Comparison principle to the infinity Laplacian equation with lower term
DOI: 10.54647/mathematics11292 74 Downloads 9514 Views
Author(s)
Abstract
In this paper, we establish the comparison principles via the perturbation method for the equation 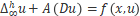
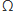
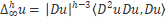
where 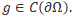
Keywords
infinity Laplacian; viscosity solutions; comparison principle; stability.
Cite this paper
Cuicui Li, Fang Liu,
Comparison principle to the infinity Laplacian equation with lower term
, SCIREA Journal of Mathematics.
Volume 6, Issue 5, October 2021 | PP. 51-62.
10.54647/mathematics11292
References
| [ 1 ] | E. Abderrahim, D. Xavier, L. Zakariaa and L. Olivier, Nonlocal infinity Laplacian equation on graphs with applications in image processing and machine learning, Mathematics and Computers in Simulation, 102(2014), 153-163. |
| [ 2 ] | G. Aronsson, Extension of functions satisfying Lipschitz conditions, Ark. Mat. 6(1967), 551-561. |
| [ 3 ] | G. Aronsson, M. G. Crandall, P. Juutinen, A tour of the theory of absolutely minimizing functions, Bull. Amer. Math. Soc. 41(2004), 439-505. |
| [ 4 ] | S. Armstrong, C. Smart, An easy proof Jensen’s theorem on the uniqueness of infinity harmonic functions. Calc. Var. Partial Differ. Equ. 37(2010), 381-384. |
| [ 5 ] | T. Bhattacharya, A. Mohammed, On solutions to Dirichlet problems involving the infinity-Laplacian, Adv. Calc. Var. 4(2011), 445-487. |
| [ 6 ] | V. Caselles, J. M. Morel and C. Sbert, An axiomatic approach to image interpolation, IEEE Trans. Image Process, 7(1998), 376-386. |
| [ 7 ] | M. G. Crandall, A visit with the ∞-Laplace equation, in Calculus of Variations and Nonlinear Partial Differential Equations, Lecture Notes in Math. 1927, pp. 75-122, Springer, Berlin, 2008. |
| [ 8 ] | M. G. Crandall, L. C. Evans and R. F. Gariepy, Optimal Lipschitz extensions and the infinity-Laplacian, Calc. Var. Partial Differ. Equ. 13(2001), 123-139. |
| [ 9 ] | M. G. Crandall, P. L. Lions, Viscosity solutions and Hamilton-Jacobi equations. Trans. Am. Math. Soc. 277(1983), 1-42. |
| [ 10 ] | M. G. Crandall, L. C. Evans, P. L. Lions, Some properties of viscosity solutions of Hamilton-Jacobi equations. Trans. Am. Math. Soc. 282(1984), 487-502. |
| [ 11 ] | M. G. Crandall, H. Ishii, P. L. Lions, User’s guide to viscosity solutions of second order partial differential equations, Bull. Amer. Math. Soc. (N. S.) 27(1992), 1-67. |
| [ 12 ] | L. C. Evansand W.Gangbo, Differential equations methods for the Monge-Kantorovich mass transfer problem, Mem. Amer. Math. Soc., 137 (1999) no.653, viii+66 pp. |
| [ 13 ] | A. Elmoataz, M. Toutain, D. Tenbrinck, On the p-Laplacian and ∞-Laplacian on graphs with applications in image and data processing, SIAM J. Imaging Sciences, 8(4) (2015), 2412-2451. |
| [ 14 ] | J. Garcia-Azorero, J. J. Manfredi, I. Peral and J. D. Rossi, The Neumann problem for the ∞−Laplacian and the Monge-Kantorovich mass transfer problem, Nonlinear Analysis: Theory Methods & Applications, 66 (2007), 349–366. |
| [ 15 ] | F. Liu, An inhomogeneous evolution equation involving the normalized infinity Laplacian with a transport term, Commun Pure Appl Anal, 17(6) (2018), 2395-2421. |
| [ 16 ] | F. Liu, F. Jiang, Parabolic Biased Infinity Laplacian Equation Related to the Biased Tug-of-War, Advanced Nonlinear Studies, 19(1) (2019), 89-112. |
| [ 17 ] | F. Liu, X.P. Yang, Solutions to an inhomogeneous equation involving infinity-Laplacian, Nonlinear Analysis: Theory, Methods & Applications, 75 (2012), 5693-5701. |
| [ 18 ] | R. López-Soriano, J. C. Navarro-Climent and J. D. Rossi, The infinity Laplacian with a transport term. J. Math. Anal. Appl. 398(2013), 752-765. |
| [ 19 ] | G. Lu, P. Wang, A PDE perspective of the normalized infinity Laplacian, Comm. Part. Diff. Eqns. 33(10) (2008), 1788-1817. |
| [ 20 ] | G. Lu, P. Wang, Inhomogeneous infinity Laplace equation. Adv. Math. 217(2008), 1838-1868. |
| [ 21 ] | K. Nyström, M. Parviainen, Tug-of-war, market manipulation, and option pricing. Math. Finance, 27(2017), 279-312. |
| [ 22 ] | Y. Peres, G. Pete, S. Somersille, Biased tug-of-war, the biased infinity Laplacian, and comparison with exponential cones, Calc. Var. PDE. 38(3-4) (2010), 541-564. |
| [ 23 ] | Y. Peres, O. Schramm, S. Sheffield, D. Wilson, Tug-of-war and the infinity Laplacian, J. Amer. Math. Soc. 22(1) (2009), 167-210. |
| [ 24 ] | J. D. Rossi, Tug-of-war games and PDEs, Proc. Roy. Soc. Edinburgh Sect. A 141(2) (2011), 319-369. |


